Class 10: Circles – Lecture Notes (II) – ICSE / ISC / CBSE Mathematics Portal for K12 Students

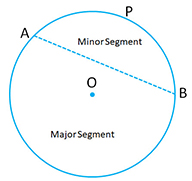
c20 Arc and it’s types:

An arc is any part of the circumference.
A chord divides the circumference of a circle in two parts or two arcs.
The arc which is less than the semi-circle is called minor arc while the one that is larger then than the semi-circle is called major arc.
A segment is the part of the circle bounded by an arc and a chord.

Theorem 9: The angle which an arc of a circle subtends at the center is double that which it subtends on any point of the part of the circumference.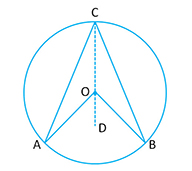

Given: $O$ is the center of the given circle. $\widehat{APB}$ subtends $\angle AOB$ at the center and $\angle ACB$ at a point $C$ on the circumference.

To Prove: $\angle AOB = 2 \angle ACB$

Proof: In $\triangle AOC$ ,

$OA = OC$ (Radius of the same circle)

Therefore $\angle OAC = \angle OCA$ (angle opposite to the equal sides of a triangle are equal)

$\angle AOD = \angle OAC + \angle OCA$ (exterior angle of a $\triangle =$ sum of the opposite interior angles)

$= \angle OCA + \angle OCA$

$= 2 \angle OCA$

Similarly in $\triangle BOC, \angle BOD = 2 \angle OCB$

Hence $\angle AOB = \angle AOD + \angle BOD$

$= 2 \angle OCA + 2 \angle OCB$

$= 2 ( \angle OCA + \angle OCB)$

$= 2 \angle ACB$ .

Hence Proved.

Similarly, if you look at the following figures you will see that:

i) $\angle AOB = 2 \angle APB$

ii) $Reflex \ \angle AOB = 2 \angle APB$

Theorem 10: Angles in the same segment of a circle are equal.

Given: A center with center $O$ . $\angle ACB \ and \ \angle ADB$ are in the same segment of the circle.

To Prove: $\angle ACB = \angle ADB$

Proof: From Theorem 9, we know that

$\angle AOB = 2 \angle ACB$ (angle at the center is twice the angle at the remaining circumference)

Similarly $\angle AOB = 2 \angle ADB$ (angle at the center is twice the angle at the remaining circumference)

This implies that $\angle ACB = \angle ADB$ .

Hence Proved.

Similarly, in the adjoining figure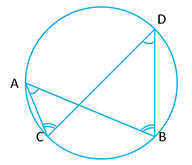

i) $\angle DAB = \angle DCB$ (Angles in the same segment)

ii) $\angle ADC = \angle ABC$ (Angles in the same segment)

Theorem 11: The angle in a semi circle is a right angle.

Given: A circle with center $O$ . $AB$ is the diameter and $\angle ACB$ is the angle of semi- circle.

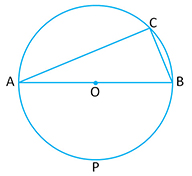
To Prove: $\angle ACB = 90^o$

Proof: $\angle AOB = 2 \angle ACB$ (angle at the center is twice the angle at the remaining circumference)

Given $\angle AOB = 180^o$ (straight line)

$\Rightarrow 180^o=2 \angle ACB$

$\Rightarrow \angle ACB = 90^o$ .

Hence Proved.

Cyclic Properties

Theorem 12: The opposite angles of a cyclic quadrilateral inscribed in a circle are supplementary.

Given: A circle with center $O$ . A quadrilateral $ABCD$ inscribed in a circle.

To Prove: $\angle ABC + \angle ADC = 180^o \ and \ \angle BAD + \angle BCD = 180^o$

Proof: $\angle AOC = 2 \angle ADC$ (angle at the center is twice the angle at the remaining circumference)

$\displaystyle \Rightarrow \angle ADC = \frac{1}{2} \angle AOC$

$\displaystyle \text{ Similarly } \angle ABC = \frac{1}{2} \text{ reflex } \angle AOC$

Adding the two

$\displaystyle \angle ABC + \angle ADC = \frac{1}{2} \angle AOC + \frac{1}{2} \text{ reflex } \angle AOC$

$\displaystyle = \frac{1}{2} (\angle AOC + \text{ reflex } \angle AOC) = \frac{1}{2} \times 360^o = 180^o$

$\displaystyle (\text{ Reflex } \angle AOC + \angle AOC = 360^o)$

Similarly we can prove that $\displaystyle \angle BAD + \angle BCD = 180^o$ .

Hence Proved.

Note:

If the opposite angles of a quadrilateral are supplementary, then the quadrilateral is cyclic
The angle in the major segment is acute and the angle in the minor segment is obtuse. i.e. $\angle ABC < 90^o$ while $\angle ADC > 90^o$