Question 1: What is the least number of solid metallic spheres, each of diameter, that should be melted and recast to form a solid metal cone whose height is
and diameter
?
Answer:
Radius of Sphere cm
Metal cone: Height cm, Radius
cm
Let the number of cones melted
Therefore
Question 2: A largest sphere is to be carved out of a right circular cylinder of radius and height
. Find the volume of the sphere. (Answer correct to the nearest integer).
Answer:
Cylinder: Radius cm, Height
cm
Radius of sphere will be cm
Volume of the largest sphere
Question 3: A right circular cylinder having diameter and height
is full of ice-cream. The ice-cream is to be filled in identical cones of height
and diameter
having a hemi-spherical shape on the top. Find the number of cones required.
Answer:h
Cylinder: Radius cm, Height
cm
Volume of ice-cream cone
Volume of cylinder
Therefore the number of ice-cream cones
Question 4: A solid is in the form of a cone standing on a hemi-sphere with both their radii being equal to and the height of cone is equal to its radius. Find, in terms of
, the volume of the solid.
Answer:
Radius cm
Volume
Question 5: The diameter of a sphere is . It is melted and drawn into a wire of diameter
. Find the length of the wire.
Answer:
Sphere: Radius cm
Wire (cylinder): Radius cm, Length
cm
Volume of sphere Volume of wire
Question 6: Determine the ratio of the volume of a cube to that of a sphere which will exactly fit inside the cube.
Answer:
Let radius of sphere be . The the side of the cube would be
Volume of sphere
Volume of cube
Hence Ratio
Question 7: An iron pole consisting of a cylindrical portion high and of base diameter
is surmounted by a cone
high. Find the mass of the pole, given that
of iron 355 has
of mass (approx.) (Take
)
Answer:
Cylinder (Iron Pole): Height cm, Radius
cm
Cone: Height cm
Volume of the structure
Density
Therefore Weight
Question 8: When a metal cube is completely submerged in water contained in a cylindrical vessel with diameter , the level of water rises by
. Find : (i) the length of the edge of the cube, (ii) the total surface area of the cube.
Answer:
Let side of the cube
Cylinder: Radius cm
Increase in height
cm
i) Therefore
Therefore cm
ii) Surface are of the cube
Question 9: In the following diagram a rectangular platform with a semi-circular end on one side is long from one end to the other end. If the length of the half circumference is
, find the cost of constructing the platform,
high at the rate of
.
Answer:
Please refer to the diagram
m
Therefore length of the rectangle m
Volume of rectangle
Volume of semi circle
Total volume
Total cost Rs.
Question 10: The cross-section of a tunnel is a square of side surmounted by a semicircle as shown in the adjoining figure. The tunnel ii
long. Calculate: (i) volume, (ii) the surface area of the tunnel (excluding the floor) and (iii) its floor area.
Answer:
Please refer to the diagram
i) Volume Cross Section Area
Length
ii) Surface area
iii) Floor area
Question 11: A cylindrical water tank of diameter and height
is being fed by a Pipe of diameter
through which water flows at the rate of
. Calculate, in minutes, the time it takes to fill the tank.
Answer:
Water tank: Radius m, Height
m
Pipe: Radius cm
Flow of water m/s
Volume of water tank
Time to fill the tank
seconds or
minutes
Question 12: Water flows, at 9 km Per hour, through a cylindrical pipe of cross-sectional area . If this water is collected into a rectangular cistern of dimensions
: calculate the rise in level in the cistern in 1 hour 15 minutes.
Answer:
Rate of flow km/hr
m/s
Volume of Cistern
Total volume of flow in hour
minutes
Height of water
Question 13: The given figure shows the cross-section of a cone, a cylinder and a hemisphere all with the same diameter 10 cm, and the other dimensions are as shown. Calculate: (a) the total surface area, (b) the total volume of the solid and (c) the density of the material if its total weight is .
Answer: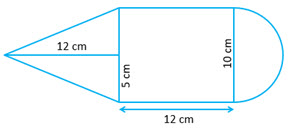
i) Total Surface Area
Since
ii) Total volume
iii) Density
Question 14: A solid, consisting of a right circular cone standing on a hemisphere, is placed upright in a right circular cylinder, full of water, and touches the bottom. Find the volume of water left in the cylinder, having given that the radius of the cylinder is and its height is
, the radius of the hemisphere is 2 cm and the height of cone is
. Give your answer to the nearest cubic centimeter.
Answer:
Cylinder: Radius cm, Height
cm
Volume of cylinder
Volume of solid
Therefore volume of water left
Question 15: A metal container in the form of a cylinder is Surmounted by a hemisphere of the same radius. The internal height of the cylinder is and the internal radius is
Calculate: (i) the total area of the internal surface excluding the base; (ii) the internal volume of the container in
Answer:
i) Total internal surface area
ii) Internal volume
Question 16: An exhibition tent is in the form of a cylinder surmounted by a cone. The height of the ten above the ground is cylindrical part is and height of the m. If the diameter of the base is
, find the quantity of canvas required to make the tent. Allow
extra for fold and for stitching. Give your answer to the nearest
Answer:
Since
Total Surface Area
Therefore canvas required
Question 17: The total surface area of a hollow cylinder, which is open from both sides, is ; area of the base ring is
and height is
. Find the thickness of the cylinder.
Answer:
Total surface area
Area of the base
Height
… … … … … i)
… … … … … ii)
Therefore from i) we get … … … … … iii)
Solving ii) and iii) we get
Hence thickness
Question 18: A test tube consists of a hemisphere and a cylinder of the same radius. The volume of the water required to fill the whole tube is
, and
of water is required to fill the tube to a level which is
below the top of the tube. Find the radius of the tube and the length of its cylindrical part.
Answer:
… … … … … i)
… … … … … ii)
From i)
Substituting in ii)
Therefore
Question 19: A solid is in the form of a right circular cone mounted on a hemisphere. The diameter of the base of the cone. which exactly coincides with hemisphere, is and its height is
. The solid is placed in a cylindrical vessel of internal radius
and height
. How much water, in
, will be required to fill the vessel completely.
Answer:
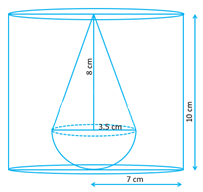 Volume of cylinder
Volume of cylinder
Volume of solid
Therefore Quantity of water required
Question 20: A cone and a cylinder have their heights in the ratio and their diameters are in the ratio
. Find the ratio between their volumes.
Answer:
Let height of the cone
Therefore the height of the cylinder
Let diameter of the cone
Therefore diameter of the cylinder
Ratio of volumes
Question 21: A sphere just fits in a cylindrical vessel and the height of the cylindrical vessel is the same as the height of the sphere. Show that the curved surface area of the cylinder is the same as the curved surface area of the sphere.
Answer:
Curved surface area of cylinder
Curved surface area of sphere
Therefore they are equal.
